moviesvast.blogg.se - Matrix meaning

Here all the types of Matrices are listed below.

There are many types of Matrices that we can use to represent the Various forms.

Matrix is defined as a Rectangular Arrangement of m and n Elements in such a way that, the Arrangement Consists of a m number of rows and n number of columns enclosed within the Square Brackets.

Matrices are used in many areas like Magnification, Cost Estimation or in the sales projection Etc.

Basically, Matrices show a Coefficient of Expressions or Equations in a meaningful way.

Matrix is referred to as a method by which we can solve some complex expressions easily.

Matrix (plural form, Matrices) in mathematics is the arrangement of numbers, expressions or symbols formed in a rectangular shape organized in the form of rows and columns.

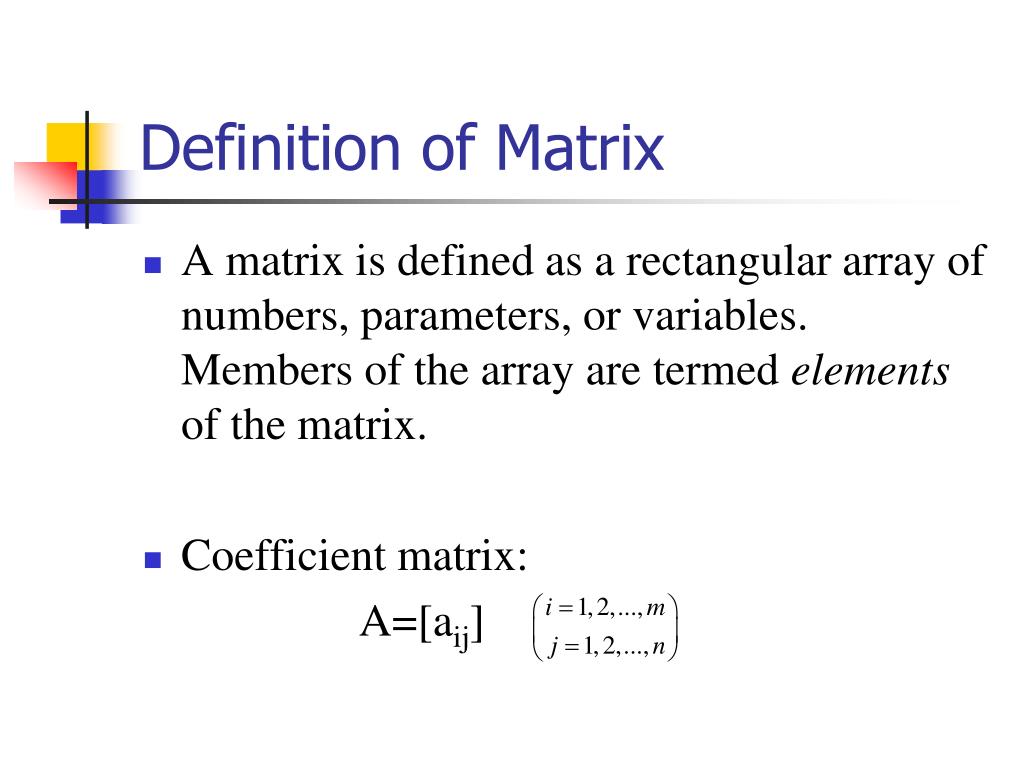
k(A+B)=k(A)+k(B) Where k is a constant and A,B are in same order of matrix.(A+B)+C=A+(B+C) where A,B and C are in the same order of matrix.Which is explained with the below shown example. Now after done with the two operations it is easy to understand the subtraction operation. Now If we do the multiplication of two matrices A and B then the resultant matrix is shown below. Now If we want to add these two matrices then we have to do addition by elements i.e first we have to add the element on 1st row and 1st column and result will be placed at 1st position of resultant matrix.Then Same thing is done with the remaining elements and their positions.Īs we do the addition of two matrices we also can do the multiplication of two matrices. Here Addition of two matrices means we have to add the elements of one matrix with the other matrix in row wise or column wise.That means we have to do addition of elements. So, We can say that it is a diagonal Matrix.Īpplications of Determinants and Matricesįollowing operations are done in the Matrix which are listed below. Here as shown in above example the matrix is of 3*3 size and 1 is written on only diagonal positions. In the Square Matrix, Elements which are halted on diagonal positions are 1 and rest elements are 0 called an Identity Matrix. Scalar MatrixĪ diagonal Matrix having the same elements on diagonal Position Then It is called as a Scalar Matrix. Zero MatrixĪ matrix having a Zero on all the positions then It is called a Zero Matrix. Diagonal MatrixĪ Matrix that has an element only on diagonal Position Then It is called a Diagonal Matrix. If the size of a Matrix is m*n then in the Square Matrix always there is m=n. Square MatrixĪ Matrix having Same Number of Rows and Same Number of Columns is called a Square Matrix. Row MatrixĪ Matrix having only One Row is called a Row Matrix. In general We can say that in the Column Matrix No. Column MatrixĪ matrix with only one Column is called a Column Matrix. Now, We will understand each type with its definition and representation.

Here all the types of Matrices are listed below. There are many types of Matrices that we can use to represent the Various forms. Matrices are shown within the or () Clauses.Throughout the whole article we will be discussing more about the matrices like Definition, Calculations, Types of Matrices and also some Examples of It. Even Matrices are also used in Cryptographical Techniques. Matrices are used in many areas like Magnification, Cost Estimation or in the sales projection Etc. Basically, Matrices show a Coefficient of Expressions or Equations in a meaningful way. Matrix is referred to as a method by which we can solve some complex expressions easily. It is important to study Matrices as it helps in projecting, operating and studying linear graphs and is also useful in representing quadratic forms. Matrices help us to study the charged resources of a circuit with voltage, resistance, amperage, etc. Keyterms: Matrices, Types, Column Matrix, Row Matrix, Square Matrix, Diagonal Matrix, Zero Matrix, Scalar Matrix, Identity Matrix